Gallery of Fourier
transform images
Part 1:
quasiperiodic point sets
ęSteffen Weber, March 1999 |
The images were
generated with my Java applet JFourier3.
click at image to see a larger image in a new window
| n-fold |
shift = 1/ n |
shift = (n-1)/(2n) |
shift = random |
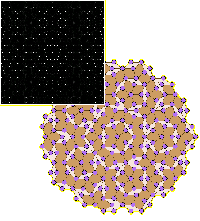
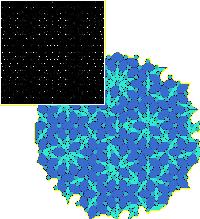
| 5-fold |
 |
 |
 |
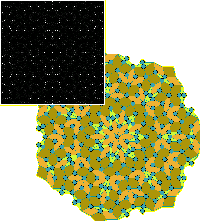
| 7-fold |
 |
 |
 |
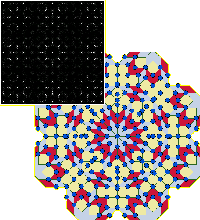
| 8-fold |
 |
 |
 |
| 9-fold |
 |
 |
 |
| 10-fold |
 |
 |
 |
| 11-fold |
 |
 |
 |
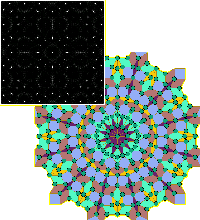
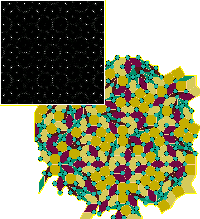
| 12-fold |
 |
 |
 |
| |
regular |
superstructure |
random
tiling |
The shift
refers to a shift of the dual grids, which generate the
tilings. Generally the tilings with a shift=(n-1)/(2n) form some
kind of superstructures, due to the existence of small periodic
tile clusters. (eg. four tiles packed periodically). The Fourier
transforms are calculated using the "atoms" at the
vertices of a tiling as point scatterers. Note that the random
tilings give a similar Fourier transform (diffraction
pattern) as the more symmetrical patterns. This is due to the long-range
orientational order of the tiles, which means that they
only occur in a few possible orientations.




















